PQ Systems: Gage Management Software
PQ Systems is now part of the Advantive family! Read announcement.
Audit-Proof Your Manufacturing Business with Gage Calibration Management Software
PQ Systems provides quality improvement solutions to help manufacturers optimize process performance, improve product quality, and mitigate supply chain risk. One of those solutions is GAGEpack, a complete gage calibration management system that provides easy access to all of your gage information including usage, calibration schedules, procedures, history, and more. Our trusted gage tracking software will help audit-proof your measurement system and give your team complete device traceability and precise calibration records.
World-Class Gage Management Software Built in the Cloud
Stay organized and maintain gage inventory, measurement systems analysis (MSA) study results, calibrations, and certificates—all in one place. Advantive’s gage management software is designed to save time, reduce variation, and optimize performance by improving the accuracy and management of your measurement devices. With the right tools to maintain a precise record of gages and events, you can quickly meet compliance standards, deliver consistent products, and make continuous improvements. Features include:
Keep gage information organized and easily accessible while ensuring precise records for internal and external device calibrations. Our highly configurable gage management software allows you to view summary information for gage inventory, historical events, and projected workload all in one place, with functionality like built-in reports, alerts, and scheduled due dates.
Maximize Your PQ Systems Software Investment
Learn to optimize your gage management system using GAGEpack with the help of our expert trainers. Whether you’re learning gage calibration best practices or tackling measurement systems analysis, GAGEpack training can be customized to help your organization achieve quality improvement success and a quick return on your investment.
Case Study
Learn how Honeybee Robotics uses PQ Systems to support its gage management system and comply with ISO and AS auditors.
What to Expect
- Free 20-minute call with a product expert
- Live demo tailored to your industry requirements
- Discover what products best fit your needs
- No games, gimmicks, or high-pressure sales pitch
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
Control Charts
Control charts: Does your data represent a process that is stable and in control?
Control charts are the best way to learn how a process is running. They are used to reduce the chance of making one of two kinds of mistakes:
- Overcontrolling
Also called a Type I error, this refers to adjusting the process when nothing out of the ordinary has occurred. - Undercontrolling
Also called a Type II error, this refers to the failure to adjust the process when something out of the ordinary has occurred
If you are not sure which chart to use for your data, review the brief descriptions on data analysis tools.
Frequently-asked questions about control charts
- Which control chart should you use?
- Multiple control limits: A long shot, or just a bad slice?
- When should you recalculate limits?
- Testing a theory about your data
For more on control charts and how they can help you, view a 4-minute video.
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
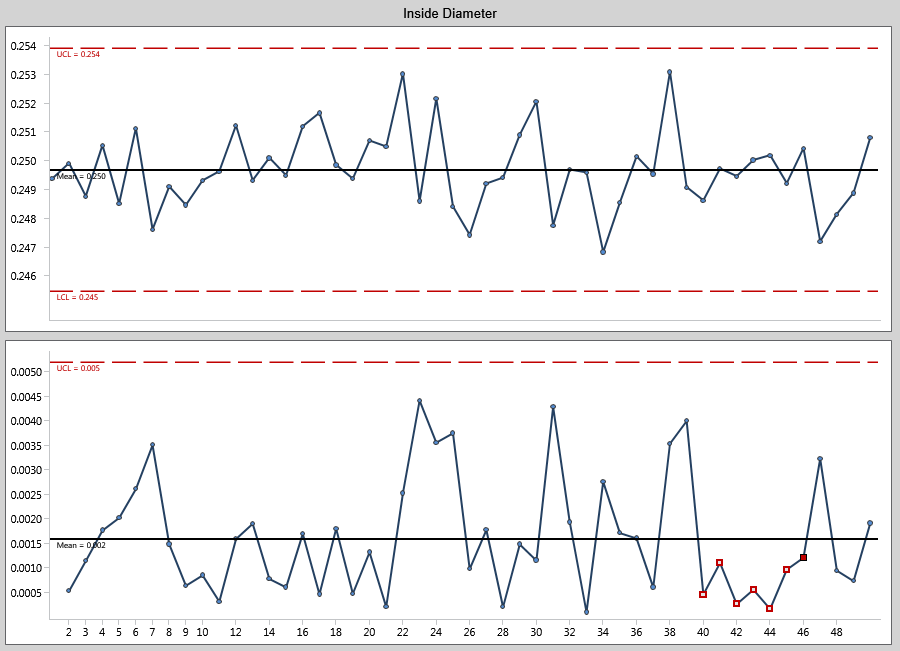
X-bar and sigma
What is it?
An X-bar and s (sigma) chart is a special purpose variation of the X-bar and R chart. Used with processes that have a subgroup size of 11 or more, X-bar and s charts show if the system is stable and predictable. They are also used to monitor the effects of process improvement theories. Instead of using subgroup range to chart variability, these charts use subgroup standard deviation. Because standard deviation uses each individual reading to calculate variability, it provides a more effective measure of the process spread. X-bar and sigma charts To create an X-bar and sigma chart using software, download a copy of SQCpack.
What does it look like?
The X-bar chart, on top, shows the mean or average of each subgroup. It is used to analyze central location. The sigma chart, on the bottom, shows how the data is spread and used to study system variability.
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
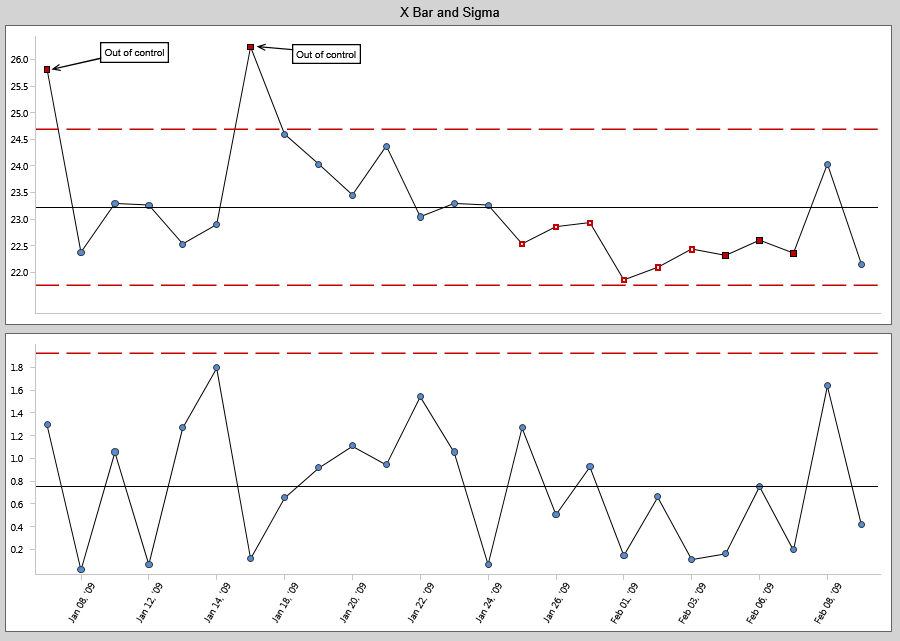
X-MR Chart
What is it?
An individuals and moving range (X-MR) chart is a pair of control charts for processes with a subgroup size of one. Used to determine if a process is stable and predictable, it creates a picture of how the system changes over time. The individual (X) chart displays individual measurements. The moving range (MR) chart shows variability between one data point and the next. Individuals and moving range charts are also used to monitor the effects of process improvement theories.
What does it look like?
The individuals chart, on top, shows each reading. It is used to analyze central location. The moving range chart, on the bottom, shows the difference between consecutive readings. It is used to study system variability.
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
X-MR Chart
What is it?
An individuals and moving range (X-MR) chart is a pair of control charts for processes with a subgroup size of one. Used to determine if a process is stable and predictable, it creates a picture of how the system changes over time. The individual (X) chart displays individual measurements. The moving range (MR) chart shows variability between one data point and the next. Individuals and moving range charts are also used to monitor the effects of process improvement theories.
What does it look like?
The individuals chart, on top, shows each reading. It is used to analyze central location. The moving range chart, on the bottom, shows the difference between consecutive readings. It is used to study system variability.
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
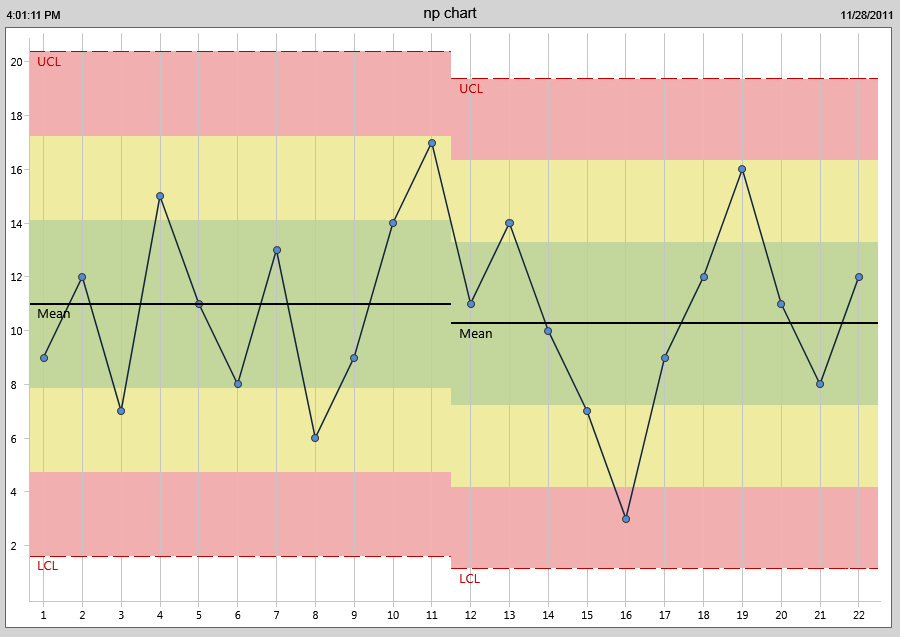
np-chart
What is it?
An np-chart is an attributes control chart used with data collected in subgroups that are the same size. Np-charts show how the process, measured by the number of nonconforming items it produces, changes over time. The process attribute (or characteristic) is always described in a yes/no, pass/fail, go/no go form. For example, the number of incomplete accident reports in a constant daily sample of five would be plotted on an np-chart. Np-charts are used to determine if the process is stable and predictable, as well as to monitor the effects of process improvement theories. Np-charts can be created using software programs like SQCpack.
What does it look like?
The np-chart shows the number of nonconforming units in subgroups of set sizes.
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
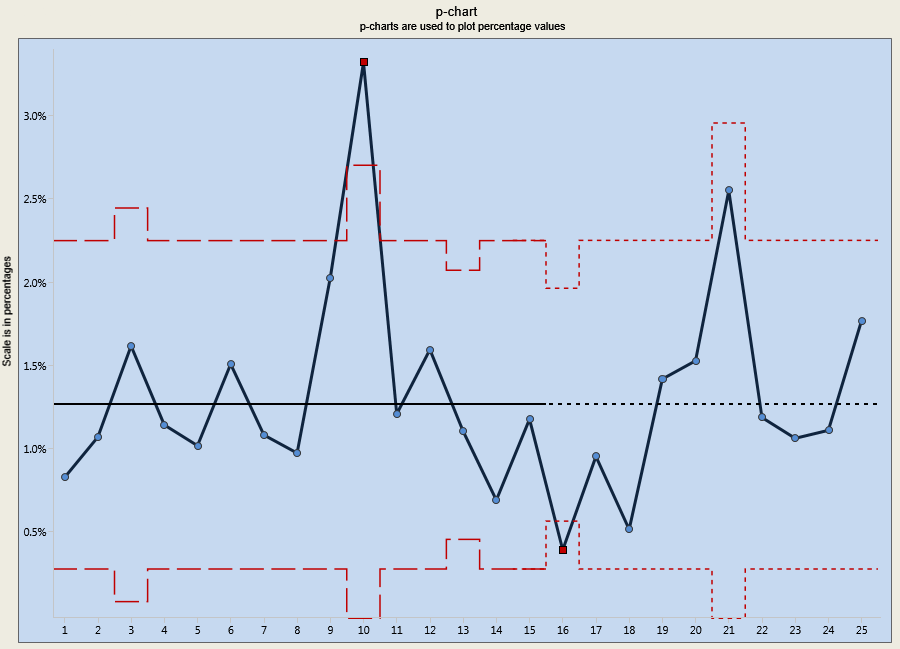
p-chart
What is it?
A p-chart is an attributes control chart used with data collected in subgroups of varying sizes. Because the subgroup size can vary, it shows a proportion on nonconforming items rather than the actual count. P-charts show how the process changes over time. The process attribute (or characteristic) is always described in a yes/no, pass/fail, go/no go form. For example, use a p-chart to plot the proportion of incomplete insurance claim forms received weekly. The subgroup would vary, depending on the total number of claims each week. P-charts are used to determine if the process is stable and predictable, as well as to monitor the effects of process improvement theories. P-charts can be created using software programs like SQCpack.
What does it look like?
The p-chart shows the proportion of nonconforming units in subgroups of varying sizes.
When is it used?
Use a p-chart when you can answer “yes” to all these questions:
1. Do you need to assess system stability?
2. Does the data consist of counts that can be converted to proportions?
3. Are there only two outcomes to any given check?
4. Has the characteristic being charted been operationally defined prior to data collection?
5. Is the time order of subgroups preserved?
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
c-chart
What is it?
A c-chart is an attributes control chart used with data collected in subgroups that are the same size. C-charts show how the process, measured by the number of nonconformities per item or group of items, changes over time. Nonconformities are defects or occurrences found in the sampled subgroup. They can be described as any characteristic that is present but should not be, or any characteristic that is not present but should be. For example a scratch, dent, bubble, blemish, missing button, and a tear would all be nonconformities. C-charts are used to determine if the process is stable and predictable, as well as to monitor the effects of process improvement theories. C-charts can be created using software products like SQCpack.
What does it look like?
The c-chart shows the number of nonconformities in subgroups of equal size.
Quality Advisor
A free online reference for statistical process control, process capability analysis, measurement systems analysis,
control chart interpretation, and other quality metrics.
SPC DEMO
Don’t miss out! Book a demo of our specialized SPC software and unlock immediate improvements in your processes.
u-chart
What is it?
A u-chart is an attributes control chart used with data collected in subgroups of varying sizes. U-charts show how the process, measured by the number of nonconformities per item or group of items, changes over time. Nonconformities are defects or occurrences found in the sampled subgroup. They can be described as any characteristic that is present but should not be, or any characteristic that is not present but should be. For example, a scratch, dent, bubble, blemish, missing button, and a tear are all nonconformities. U-charts are used to determine if the process is stable and predictable, as well as to monitor the effects of process improvement theories. U-charts can be created using software programs like SQCpack.
What does it look like?
The u-chart shows the proportion of nonconformities units in subgroups of varying sizes.
